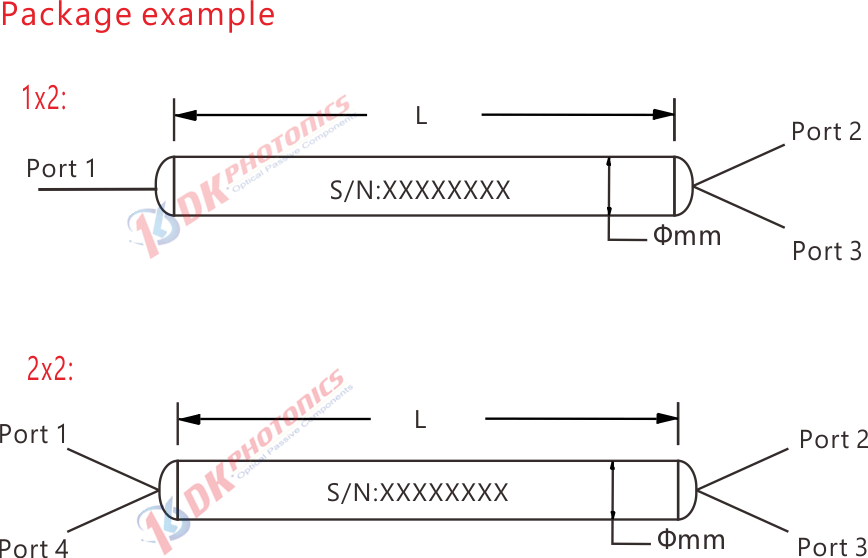
<h3 style=”text-align: center;”>Fused biconical fiber coupler</h3>
<strong>1. Definition</strong>
Fused biconical fiber coupler (FBT coupler) is a kind of optical passive device which can couple the optical signal in the coupling region of special structure and redistribute it. In the early stage, it was mostly used to take out a certain power in the transmission trunk road for monitoring. With the rapid development of optical fiber technology, optical fiber coupler has been gradually developed1 × 2 / 2 × 2 / 1 × 3 or 3 × 3 and other coupling types, as well as a variety of different coupling ratio applications.
<strong>2. Characteristic</strong>

1). A beam of light can be divided into two or more beams according to a certain power ratio.

2). Wide working wavelength bandwidth, low additional loss, high stability and reliability.

3).Made by melting taper technology, it provides a variety of wavelength options in the wavelength range of 405 nm-2050 nm.

<strong>3．Description</strong>
<strong>1). Fused taper coupler</strong>
The fused taper coupler is composed of two parallel optical fibers The optical fibers are wound together in the high temperature environment, the optical fibers are gradually melted and stretched at the same time, so that the fiber cores of two optical fibers are gradually close to a certain distance. The area which these two optical fibers winding and fusion stretching is called Coupling Region, whose structure is shown in Figure 1:

<img class=”aligncenter wp-image-7744″ src=”https://www.dkphotonics.com/wp-content/uploads/schematic-diagram-of-regional-structure-and-optical-coupling-distribution-of-fused-taper-1024×445.png” alt=”” width=”800″ height=”348″ />
Figure 1. Schematic diagram of regional structure and optical coupling distribution of fused taper(Red is fiber core, Purple is cladding, Green and Blue are light).

The length L of the coupling region determines the coupling ratio of these two fibers. In the manufacturing process, the light wave is continuously input at the pin port, all the output power of each output port is monitored in real time. When the designed coupling ratio is reached, it would stop stretching. Such a coupler is essentially one optical fiber with two very close core and this process is called FBT(FBT,Fused Biconical Taper).
<strong>2). Theory</strong>
The intensity distribution of light passing through an optical fiber is basically a Gaussian distribution. That is, the strength is greatest at the center, the energy decreases as the center approaches the core/cladding interface. It is noteworthy that the tail energy of the Gaussian distribution extends slightly beyond the boundary between the fiber core and cladding, and the light wave of this tail energy is called evanescent wave. Figure 2 shows the energy distribution of light wave in the cross section of optical fiber. The vertical dotted line represents the fiber core / cladding boundary, and the red part is the evanescent wave energy.

<img class=”aligncenter wp-image-7751″ src=”https://www.dkphotonics.com/wp-content/uploads/the-energy-distribution-of-light-transmitted-in-optical-fiber-300×166.png” alt=”” width=”380″ height=”211″ />
Figure 2 The energy distribution of light transmitted in optical fiber (The orange part represents the energy of evanescent wave.

During process of FBT, the cores of two parallel fibers are so close that evanescent waves can “leak” from one core to the other. The degree of energy exchange in the coupling region mainly depends on the spacing(d) between the cores and the length(l) of the coupling region. It can be found in Figure 1 that if the coupling length is long enough, the energy can be completely coupled from one fiber to another. If it is longer, the coupling process will continue to transfer energy back to the original fiber. By selecting the appropriate length, any given power transfer ratio can be realized. This is why we can make 50 / 50 or 10 / 90 coupling ratio by controlling the process.

<strong>3). Examples</strong>

<img class=”aligncenter wp-image-7709″ src=”https://www.dkphotonics.com/wp-content/uploads/2×2-port-structure-50-50-tapered-coupler-300×80.png” alt=”” width=”650″ height=”173″ />
Figure 3. 2 × 2-port structure 50 / 50 tapered coupler.

As show in the Figure 3, it is fused taper coupler with 50/50 coupling ratio. Assuming that we input 1 mW and 1550 nm light to port 1 and port 4, how much optical power can we measure at output ports 2 and 3 respectively? Obviously, we will measure the power of 1 mW at each output port, and the light at each input port is divided into 50 / 50 equal parts.
Suppose we input 1 mW to port 1 and 2 mW to port 4. How much power can output ports 2 and 3 measure respectively? According to the previous conclusion, the input optical energy of each path is divided into two equal parts, so now we measure the power of 1.5 MW at each output port (port 1 provides 0.5 mW and port 4 provides 1 mW).
Suppose that the port 4 is now damaged (Generally, the three port devices are made into four ports first, and then the optical fiber of the four ports is broken and roughened to avoid the reflection echo generated by the smooth section), it becomes a 1 × 2 coupler. If we input 2 mW at port 1, it is easy to know that we will finally measure 1 mW at ports 2 and 3 respectively.

<strong>4). Reciprocity of tapered couplers</strong>
At a standard 2 × 2 coupler with 50 / 50 coupling ratio, if we flip the light direction of input and output, because of the symmetry of its structure, it is easy to understand that its forward and reverse transmission has the same properties. However, when using 1 × 2 coupler, people sometimes have doubts, because the obvious asymmetry of the device will give people an illusion that the working principle of its reverse transmission will be different from that of forward transmission.
Suppose that the port 4 is now damaged for the 2 × 2 coupler, if the light is input from the original two “output” ports 2 and 3, will the light come out of port 1 100%? Of course not. Light also wants to come out of the damaged port 4. Therefore, if we only input 1 mW from port 2, only 0.5 mW is output from port 1. Or we input 1 mW to port 2 and 2 mW to port 3, there would be 1.5 mW output from port 1 (port 2 provides 0.5 mW and port 3 provides 1 mW). This energy distribution is consistent with forward transmission. Therefore, the tapered coupler has reciprocity, and the forward and backward transmission has the same properties.
So, the 1 × 2 coupler is essentially a 2 × 2 coupler, just one the fiber was cut (To reduce reflection from the section).

<strong>5). Key parameter</strong>
<strong>Insertion Loss</strong>
The insertion loss is defined as the ratio of the input power to the output power at one of the output legs of the coupler (signal or tap). Insertion loss is always specified in decibels (dB). It is generally defined using the equation below:

<img class=”aligncenter size-medium wp-image-7725″ src=”https://www.dkphotonics.com/wp-content/uploads/IL-function-300×44.png” alt=”” width=”300″ height=”44″ />

where Pin and Pout are the input and output powers (in mW). For our 1×2 couplers, the insertion loss specification is provided for both signal and tap outputs; our specifications always list insertion loss for the signal output first. To define the insertion loss for a specific output (port 2 or port 3), the equation is rewritten as:

<img class=”aligncenter wp-image-7722″ src=”https://www.dkphotonics.com/wp-content/uploads/IL-1-2-300×34.png” alt=”” width=”420″ height=”48″ />

&nbsp;

<img class=”aligncenter wp-image-7723″ src=”https://www.dkphotonics.com/wp-content/uploads/IL-1-3-300×34.png” alt=”” width=”420″ height=”48″ />

Insertion loss inherently includes both coupling (e.g., light transferred to the other output leg) and excess loss (e.g., light lost from the coupler) effects. The maximum allowed insertion loss for each output, signal and tap, are both specified. Because the insertion loss in each output is correlated to light coupled to the other output, no coupler will ever have the maximum insertion loss in both outputs simultaneously.

<strong>Calculating Insertion Loss using Power Expressed in dBm</strong>
Insertion loss can also be easily calculated with the power expressed in units of dBm. The equation below shows the relationship between power expressed in mW and dBm:

<img class=”aligncenter wp-image-7736″ src=”https://www.dkphotonics.com/wp-content/uploads/P-dBm-300×29.png” alt=”” width=”260″ height=”25″ />

Then, the insertion loss in dB can be calculated as follows:

<img class=”aligncenter size-medium wp-image-7725″ src=”https://www.dkphotonics.com/wp-content/uploads/IL-function-300×44.png” alt=”” width=”300″ height=”44″ />

<strong>Excess Loss</strong>
Excess Loss refers to the overall energy transmission loss of the optical fiber coupler. It is defined in dB is determined by the ratio of the total input power to the total output power:

<img class=”aligncenter wp-image-7717″ src=”https://www.dkphotonics.com/wp-content/uploads/Excess-Loss-300×32.png” alt=”” width=”450″ height=”48″ />

Pport1 is the input power at port 1 and Pport2+Pport3 is the total output power from Ports 2 and 3. All powers are expressed in mW.

<strong>Polarization Dependent Loss (PDL)</strong>
The polarization dependent loss is defined as the ratio of the maximum and minimum transmissions due to polarization states in couplers. This specification pertains only to couplers not designed for maintaining polarization. PDL is always specified in decibels (dB), and can be calculated with the following equation:

<img class=”aligncenter wp-image-7737″ src=”https://www.dkphotonics.com/wp-content/uploads/pdl-300×31.png” alt=”” width=”450″ height=”46″ />

where Pmax is the maximum power able to be transmitted through the coupler when scanning across all possible polarization states. Pmin is the minimum transmission across those same states.

<strong>Return Loss (RL)</strong>

The return loss refers to the fraction of input light that is lost in the internally terminated fiber end within the coupler housing when any port is used as the input. It can be calculated in units of dB using the following equation:

<img class=”aligncenter size-medium wp-image-7739″ src=”https://www.dkphotonics.com/wp-content/uploads/return-loss-300×46.png” alt=”” width=”300″ height=”46″ />

where P and Pb are the optical powers (in mW) in any port and the internally terminated fiber, respectively. This output is the result of back reflection at the junction of the legs of the coupler.

<strong>Directivity</strong>
Crosstalk between two output (port 2 and port 2) is referred to as directivity:

<img class=”aligncenter size-medium wp-image-7716″ src=”https://www.dkphotonics.com/wp-content/uploads/directivity-300×47.png” alt=”” width=”300″ height=”47″ />

The light reflected from the coupler must be minimal so as not to interfere with the measurement signal. The sources of interference are kept as small as possible. This includes assembly and packaging of the connectors.
Usually, the return loss and directivity of the taper coupler are excellent. But if the coupler has a connector, the end face of the connector must be very clean, otherwise it will affect the return loss and directivity.

<strong>Coupling Ratio</strong>
Insertion loss (in dB) is the ratio of the input power to the output power from each leg of the coupler as a function of wavelength. It captures both the coupling ratio and the excess loss. The coupling ratio is calculated from the measured insertion loss. As show in the Figure 4, Coupling ratio (in %) is the ratio of the optical power from each output port (ports 2 and 3) to the sum of the total power of both output ports as a function of wavelength. Path A represents light traveling from port 1 to port 2 while Path B represents light traveling from port 1 to port 3.

<img class=”aligncenter wp-image-7713″ src=”https://www.dkphotonics.com/wp-content/uploads/coupling-ratio-1024×255.png” alt=”” width=”850″ height=”212″ />
Figure 4. A graphical representation of the coupling ratio calculation.

<strong>Uniformity</strong>
The uniformity is also calculated from the measured insertion loss. Uniformity is the variation (in dB) of the insertion loss over the bandwidth. It is a measure of how evenly the insertion loss is distributed over the spectral range. Because different wavelengths of light in the optical fiber loss value is different, so the uniformity of optical fiber coupler is defined as: In the whole working wavelength range, the ratio of minimum output optical power Pmin Out and maximum output optical power Pmax Out, this indicates the stability of the output optical power when the input power changes within the working wavelength range. The smaller the uniformity value, the better (Note: Generally, the calculation result is negative, but the negative sign is often omitted in practice.).